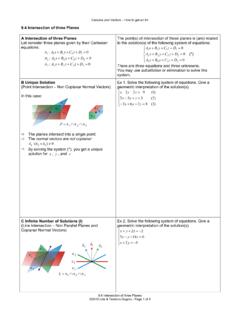
1.3 Limits (II) A. Piecewise-defined Functions

1 Limits (II) A. Piecewise-defined Functions Let consider thatis a Piecewise-defined function: )(xf >=<=axxhaxcaxxgxf),(,),()( Then: )(lim)(limxgxfaxax =and )(lim)(limxhxfaxax++ =Ex: >+ =0,10,1)(2xxxxxf. Find . )(lim0xfx B. Algebraic Identities The following algebraic identities may be useful to find algebraically the limit of a function: oddnbbabaabababbabaababababbaabababababa babababababababannnnnnnnnnnn),..)(()..)( ())(())(())(())((12321123213223442233223 322 + +=+++++ = +++ = + +=+++ = + = C. Rational Functions Consider a rational function in the form: 0)(,)()()( =xQxQxPxfwhereandare polynomial Functions . If)(xP)(xQax=is a common zero ofandthen the limit leads to the indeterminative )(xP)(xQ)(limxfax 00. This indeterminative may be eliminated by dividing bothandby the common factor)(xP)(xQax . Ex: Find 11lim21 xxx. D. Conjugate Radicals In same cases, to eliminate an indeterminative of the form 00we multiply both the numerator and denominator by a conjugate radical in order to cancel out a common zero.

2 The conjugate radical of ba is ba+and bababa =+ ))(( Ex: Find 11lim1 xxx. E. Change of Variable A change of the independent variable might simplify the process of evaluating Limits . By changing the independent variable we must change accordingly the numberax= where we calculate the limit. Ex: Find 11lim31 xxx. F. Squeeze Theorem Let assume that)()()(xhxfxg on an open interval containing the numberax=and .Then: Lxhxgaxax== )(lim)(limLxfax= )(limEx: Find xxx1sinlim0. G. Fundamental Limits of Calculus Calculus is based on 3 fundamental Limits : 1. Power Functions (case00) = + ,1)1(lim0xxx Ex: Find xxx11lim0 + . 2. Exponential Functions (case ) 1exxx=+ 10)1(lim Ex: Findxxx30)21(lim+ . 3. Trigonometric Functions (case 00) 1sinlim0= xxx Ex: xxx5)2sin(lim0 MCV4U | Limits | Limits (II) 2009 Teodoru Gugoiu | Page 1 of 3 A. Piecewise-defined Functions 1. Evaluate, if it exists. a) b) |1|lim1 xxxxx||lim0 c) xxx + 1|1|lim1 d) e) ||lim0xxx |1|1lim21 xxx f) xxxx)1(||lim0+ 2.

3 The Heaviside function is defined by: <=0100)(tiftiftH Evaluate, if it exits: a) b) c) )(lim0tHx )(lim0tHx+ )(lim0tHx 3. Consider the function: > <+=11111)(2xifxxifxxifxxf Find the following Limits , if they exit. a) b) c) )(lim1tfx )(lim0tfx )(lim1tfx 4. Find such that the function: c <+=0cos0sin1)(xifxcxifxxf has a limit asx approaches . 0 5. Find and bsuch that the function: a +< <+=1ln100)(xifxbxifxxifeaxfx has a limit everywhere (at any number). 6. The function is defined as the largest integer that is less than or equal to][xx. Find, if it exists: a) b) c) ][lim0xx ][lim0xx+ ][lim0xx d) e) f) ])[(lim1xxx ][lim0xxx xxx][lim0 g) integeranisnxnx][lim h) integeranisnxnx][lim+ 7. The sign function is defined by: >=< =110001)sgn(xifxifxifx Find, if it exists: a) b) c) )sgn(lim0xx )sgn(lim0xx+ )sgn(lim0xx d) e) f) |)sgn(|lim0xx |)sgn(|lim0xx )sgn(lim0xxx B.

4 Algebraic Identities C. Rational Functions 1. Evaluate. a) 24lim22+ xxx b) 12lim21 + xxxx c) 48lim232 xxx d) 3432lim223+ xxxxx e) 327lim33++ xxx f) 2211lim2 xxx g) 216)2(lim22 + xxx h) 11lim341 xxx i) xxxx110)3()3(lim + D. Conjugate Radicals 1. Evaluate. a) 24lim4 xxx b) 1lim1 xxxx c) 1326lim2 xxx d) ttt33lim0 + e) 111lim1 xxx f) + tttt111lim0 g) xxxx+ 33lim0 h) xxxxx + + 2211lim0 i) xxxx 1lim21 E. Change of Variable 1. Evaluate. a) 28lim38 xxx b) 48lim2/34 xxx F. Squeeze Theorem 1. Let. Find. =irrationalisxifrationalisxifxxf0)(2)(li m0xfx G. Fundamental Limits of Calculus 1. Find the following Limits : a) 12111lim530 + + xxx b) 54301111limxxxxx+ ++ + MCV4U | Limits | Limits (II) 2009 Teodoru Gugoiu | Page 2 of 3 2. Find the following Limits : a) xxxx/1011lim + b) xxx120)1(lim+ c) xxx10)||1(lim+ d) xxx)1ln(lim0+ e) 1ln2lim21 xxx f) xxx2)31ln(lim0 3.

5 Find the following Limits : a) xxxcos1lim0 b) 20cos1limxxx c) xxxtanlim0 d) xxx220tan)sin(lim CQ. Challenge Questions 1. The Dirichlet function is defined by =irrationalisxifrationalisxifxf01)( Find, if it exists: a) b) )(lim1xfx )(limxfx 2. Find and bsuch that a12lim0= + xbaxx. 3. If the following limit exists 233lim222 ++++ xxaaxxx find the value of and the value of the limit. a 4. Evaluate xaxx11lim30 + . 5. Find the Limits if they exist (is the largest integer that is less than or equal to][xx). a) xxx1lim0 b) xxx1lim20 6. Let xxf/1211)(+=. Determine whether exists. )(lim0xfx 7. Find the following Limits : a) 30sinlimxxxx b) xxxsin10)(coslim c) 20coslnlimxxx d) )1()1()1()1(lim0xxxxx+ ++ + A1. a) 0 b) DNE c) -1 d) 0 e) DNE f) DNE 2. a) 0 b) 1 c) DNE 3. a) DNE b) 0 c) 1 4. 2 5. 1 =aand 1=b 6. a) -1 b) 0 c) DNE d) DNE e) 0 f) DNE g) 1 nh) n 7.

6 A) -1 b) 1 c) DNE d) 1 e) 1 f) 0 C1. a) -4 b) 3 c) 3 d) 2 e) 27 f) -1/4 g) 8 h) 4/3 i) 2/9 D1. a) 4 b) -1/2 c) 1/2 d) )32/(1e) -1/2 f) -1/2 g) 3/1 h) 2i) 3 E1. a) 12 b) 3 F1. 0 G1. a) 5/3 b) 10/3 c) 2. a) b) 1 c) DNE d) 1 e) 1 f) -3/2 2e3. a) 0 b) 1/2 c) 1 d) 1 CQ1. a) DNE b) DNE 2. 4=aand 4=b 3. 15=a and 1 =L 4. 1/3 5. a) 1 b) 0 6. DNE 7. a) 1/6 b) 1 c) 1/2 d) MCV4U | Limits | Limits (II) 2009 Teodoru Gugoiu | Page 3 of 3