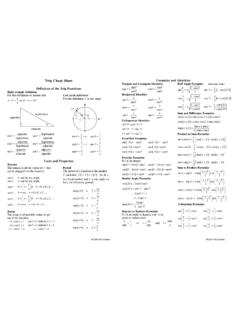
nn) (cx ncx nn) - Lamar University

1 Common Derivatives and Integrals Visit for a complete set of Calculus I & II notes. 2005 Paul Dawkins Derivatives Basic Properties/Formulas/Rules ()()( )dcf xcfxdx =, c is any constant. ()( )()() ()fxgx fxgx = ( )1nndxnxdx =, n is any number. ()0dcdx=, c is any constant. ( )fgf g fg = + (Product Rule) 2ff g fggg = (Quotient Rule) ( )()()( )()()df gxf gx g xdx = ( Chain Rule) ( )()( )()gxgxdgxdx =ee ( )()( )( )lngxdgxdxg x = Common Derivatives Polynomials ( )0dcdx= ( )1dxdx= ()dcxcdx= ( )1nndxnxdx = ( )1nndcxncxdx = Trig Functions ()sincosdxxdx= ()cossindxxdx= ()2tansecdxxdx= ()secsec tandxxxdx= ()csccsc cotdxxxdx= ()2cotcscdxxdx= Inverse Trig Functions ()121sin1dxdxx = ()121cos1dxdxx = ()121tan1dxdxx =+ ()121sec1dxdxxx = ()121csc1dxdxxx = ()121cot1dxdxx = + Exponential/Logarithm Functions ( )( )lnxxdaaadx= ( )xxddx=ee ( )()1ln,0dxxdxx=> ()1ln,0dxxdxx= ( )()1log,0lnadxxdxxa=> Hyperbolic Trig Functions ()sinhcoshdxxdx= ()coshsinhdxxdx= ()2tanhsechdxxdx= ()sechsech tanhdxxxdx= ()cschcsch cothdxxxdx= ()

2 2cothcschdxxdx= Common Derivatives and Integrals Visit for a complete set of Calculus I & II notes. 2005 Paul Dawkins Integrals Basic Properties/Formulas/Rules ( )( )cf x dxc f x dx= , c is a constant. ( ) ( )( )()f xg x dxf x dxg x dx = ( )()( ) ( )bbaaf x dxF xF bF a== where ( )( )F xf x dx= ( )()bbaacf x dxcf x dx= , c is a constant. ( ) ( )( )( )bbbaaaf xg x dxf x dxg x dx = ()0aaf x dx= ( )( )baabf x dxf x dx= ()( )()b cba acf x dxf x dxf x dx=+ ()bac dxc b a= If ( )0fx on a xb then ( )0baf x dx If ( ) ( )f x gx on a xb then ( )( )bbaaf x dxg x dx Common Integrals Polynomials dxx c= + k dxk x c= + 11,11nnx dxxc nn+=+ + 1lndxxcx= + 1lnx dxxc = + 11,11nnx dxxc nn +=+ + 11lndxax bcax ba=+++ 111pppqqqqpqqx dxxcxcpq++=+=+++ Trig Functions cossinu duu c=+ sincosu duu c= + 2sectanu duu c=+ sec tansecuu duu c=+ csc cotcscuuduu c= + 2csccotu duu c= + tanln secu duuc=+ cotln sinu duuc=+ secln sectanu duuuc= ++ ()31secsec tanln sectan2u duuuuuc=+ ++ cscln csccotu duuuc= + ()

3 31csccsc cotln csccot2u duuuuuc= + + Exponential/Logarithm Functions uuduc= + ee lnuuaa duca= + ( )lnlnu duuuu c= + ( )( )( )()22sinsincosauaubu duabubbucab= ++ ee ()1uuu duuc= + ee ()( )( )()22coscossinauaubu duabubbucab=+++ ee 1ln lnlnduucuu=+ Common Derivatives and Integrals Visit for a complete set of Calculus I & II notes. 2005 Paul Dawkins Inverse Trig Functions 1221sinuducaau =+ 112sinsin1u duuuuc =+ + 12211tanuducauaa =+ + ()11 21tantanln 12u duuuuc = ++ 12211secuducaauu a =+ 112coscos1u duuuuc = + Hyperbolic Trig Functions sinhcoshu duu c=+ sech tanhsechuu duu c= + 2sechtanhu duu c=+ coshsinhu duu c=+ csch cothcschuu duu c= + 2cschcothu duu c= + ()tanhln coshu duuc=+ 1sechtansinhu duuc =+ Miscellaneous 2211ln2uaduca ua ua+=+ 2211ln2uaducu aa ua =+ + 2222222ln22uaauduauu au c+ = ++ + ++ 2222222ln22uauaduuau ua c = + + 222221sin22uauauduauca = ++ 222122cos22uaaauau u duau uca = ++ Standard integration Techniques Note that all but the first one of these tend to be taught in a Calculus II class.

4 U Substitution Given ( )()( )baf g x g x dx then the substitution ( )u gx= will convert this into the integral, ( )()( )( )( )( )bgbagaf g x g x dxf u du = . integration by Parts The standard formulas for integration by parts are, bbbaaaudv uvvduudv uvvdu= = Choose u and dv and then compute du by differentiating u and compute v by using the fact that vdv= . Common Derivatives and Integrals Visit for a complete set of Calculus I & II notes. 2005 Paul Dawkins Trig Substitutions If the integral contains the following root use the given substitution and formula . 22222sinandcos1 sinaa bxxb == 22222secandtansec1abx axb == 22222tanandsec1 tanaa bxxb + ==+ Partial Fractions If integrating ()()PxdxQx where the degree (largest exponent) of ( )Px is smaller than the degree of ( )Qx then factor the denominator as completely as possible and find the partial fraction decomposition of the rational expression.

5 Integrate the partial fraction decomposition ( ). For each factor in the denominator we get term(s) in the decomposition according to the following table. Factor in ()Qx Term in Factor in ( )Qx Term in ax b+ Aax b+ ()kax b+ ()()122kkAAAax bax bax b++++++ 2axbx c++ 2Ax Baxbx c+++ ()2kaxbx c++ ()1122kkkAx BAx Baxbx caxbx c++++++++ Products and (some) Quotients of Trig Functions sincosnmxx dx 1. If n is odd. Strip one sine out and convert the remaining sines to cosines using 22sin1 cosxx= , then use the substitution cosux= 2. If m is odd. Strip one cosine out and convert the remaining cosines to sines using 22cos1 sinxx= , then use the substitution sinux= 3. If n and m are both odd. Use either 1. or 2. 4. If n and m are both even. U se double angle formula for sine and/or half angle formulas to reduce the integral into a form that can be integrated.

6 Tansecnmxx dx 1. If n is odd. Strip one tangent and one secant out and convert the remaining tangents to secants using 22tansec1xx= , then use the substitution secux= 2. If m is even. Strip two secants out and convert the remaining secants to tangents using 22sec1 tanxx= +, then use the substitution tanux= 3. If n i s odd and m is even. Use either 1. or 2. 4. If n is even and m is odd. Each integral will be dealt with differently. Convert Example : () ()33622coscos1 sinxxx==