Transcription of Partial derivatives and differentiability (Sect. 14.3 ...
1 Partial derivatives and differentiability (Sect. ).IPartial derivatives and functionsf:D R2 and primer on differential derivatives and :The following result holds for single variable the function f:R Ris differentiable, then f is 0[f(x+h) f(x)] = limh 0[f(x+h) f(x)h]h,=f (x) limh 0h= is, limh 0f(x+h) =f(x), sofis , the claim Iffx(x,y) andfy(x,y) exist, thenf(x,y)is continuous is derivatives and the function f:R Ris differentiable, then f is :IThis Theorem is not true forthe Partial derivatives of afunctionf:R2 exist functionsf.
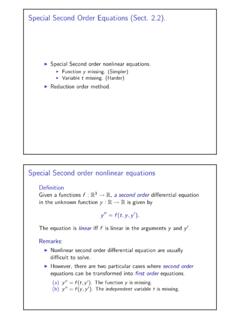
2 R2 Rsuch thatfx(x0,y0) andfy(x0,y0) existbutfis not continuous at(x0,y0).1f(x,y)CC12xyzf (0,0) = f (0,0) = 0yxRemark:This is a bad property for a differentiable derivatives and :Here is a discontinuous function at (0,0) having partialderivatives at (0,0).Example(a)Show thatfis not continuous at (0,0), wheref(x,y) = 2xyx2+y2(x,y)6= (0,0),0(x,y) = (0,0).(b)Findfx(0,0) andfy(0,0).Solution:(a)Choosing the pathx= 0 we see thatf(0,y) = 0, solimy 0f(0,y) = the pathx=ywe see thatf(x,x) = 2x2/2x2= 1, solimx 0f(x,x) = Two-PathTheorem implies thatlim(x,y) (0,0)f(x,y) does not (a)Show thatfis not continuous at (0,0), wheref(x,y) = 2xyx2+y2(x,y)6= (0,0),0(x,y) = (0,0).
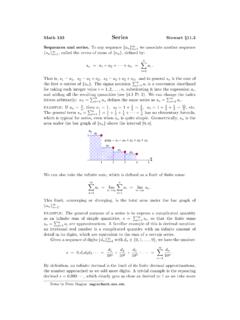
3 (b)Findfx(0,0) andfy(0,0).Solution:(b)The Partial derivatives are defined at (0,0).fx(0,0) = limh 01h[f(0 +h,0) f(0,0)]= limh 01h[0 0] = (0,0) = limh 01h[f(0,0 +h) f(0,0)]= limh 01h[0 0] = ,fx(0,0) =fy(0,0) = derivatives and differentiability (Sect. ).IPartial derivatives and functionsf:D R2 and primer on differential functionsf:D R2 :A differentiablefunctionf:R Ratx0must be approximated bya lineL(x) containingx0with slopef (x0).f ( x ) = zx0xf ( x ) L ( x )0 Line that approximatesf ( x ) at x.
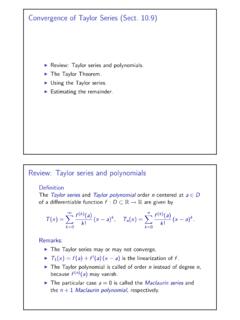
4 0 The equation of the tangent line isL(x) =f (x0) (x x0) +f(x0).The functionfis approximated by the lineLnearx0meansf(x) =L(x) + 1(x x0)with 1(x) 0 asx graph of a differentiable functionf:D R Risapproximated by a line at every point functionsf:D R2 :The idea to define differentiable functions :The graph of a differentiable functionf:D R2 Risapproximated by a plane at every point (x,y)( x , y )00 Plane that f ( x, y ) at ( x , y )approximates f ( x, y ) = zxyFunction f is differentiable at (x , y ).
5 0 00near ( 0 , 0 ).xyz1f(x,y)Plane that does notL ( x , y )approximate f (x,y)Function f is not differentiable at ( 0 , 0 ).We will show next week that the equation of the planeLisL(x,y) =fx(x0,y0) (x x0) +fy(x0,y0) (y y0) +f(x0,y0).Differentiable functionsf:D R2 a functionf:D R2 Rand an interior point (x0,y0) inD, letLbe the plane given byL(x,y) =fx(x0,y0) (x x0) +fy(x0,y0) (y y0) +f(x0,y0).The functionfis calleddifferentiable at(x0,y0)iff the functionfis approximated by the planeLnear (x0,y0), that is,f(x,y) =L(x,y) + 1(x x0) + 2(y y0)where the functions 1and 2 0 as (x,y) (x0,y0).
6 The functionfisdifferentiableifffis differentiable at everyinterior point functionsf:D R2 :Recalling that the equation for the planeLisL(x,y) =fx(x0,y0) (x x0) +fy(x0,y0) (y y0) +f(x0,y0),an equivalent expression forfbeing differentiable,f(x,y) =L(x,y) + 1(x x0) + 2(y y0),is the following:Denotez=f(x,y) andz0=f(x0,y0), andintroduce the increments z= (z z0), y= (y y0), x= (x x0);then, the equation above is z=fx(x0,y0) x+fy(x0,y0) y+ 1 x+ 2 y.(Equation used in the textbook to define a differentiable function.)
7 Partial derivatives and differentiability (Sect. ).IPartial derivatives and functionsf:D R2 and primer on differential and :The graph of adifferentiable functionf:D R2 Ris approximatedby a plane at every point (x,y)( x , y )00 Plane that f ( x, y ) at ( x , y )approximates f ( x, y ) = zxyFunction f is differentiable at (x , y ). 0 00 Remark:A simple sufficient condition on a functionf:D R2 Rguarantees thatfis differentiable:TheoremIf the Partial derivatives fxand fyof a function f:D R2 Rarecontinuous in an open region R D , then f is differentiable in R.
8 TheoremIf a function f:D R2 Ris differentiable, then f is derivatives and differentiability (Sect. ).IPartial derivatives and functionsf:D R2 and primer on differential primer on differential :Adifferential equationis an equation where the unknownis a function and the function together with its derivatives appearin the a constantk R, find all solutionsf:R Rto thedifferential equationf (x) =k f(x).Solution:Multiply the equation abovef (x) kf(x) = 0 bye kx,that is,f (x)e kx f(x)ke kx= left-hand side is a total derivative,[f(x)e kx] = solution of the equation above isf(x)e kx=c, withc ,f(x) =c primer on differential are three differential equations for functionsf:D Rn R, withn= 2,3,4, that appear in several equation: (Gravitation, electrostatics.)
9 2xf+ 2yf+ 2zf= equation: (Heat propagation, diffusion.) tf=k( 2xf+ 2yf+ 2zf).ITheWave equation: (Light, sound, gravitation.) 2tf=v( 2xf+ 2yf+ 2zf).A primer on differential thatf(x,y,z) =1 x2+y2+z2satisfies the Laplaceequation :fxx+fyy+fzz= :Recall:fx= x/(x2+y2+z2)3 ,fxx= 1(x2+y2+z2)3/2+322x2(x2+y2+z2)5 x2+y2+z2,thenfxx= 1r3+ ,fyy= 1r3+3y2r5, andfzz= 1r3+ ,fxx+fyy+fzz= 3r3+3(x2+y2+z2)r5= 3r3+3r2r5= conclude thatfxx+fyy+fzz= primer on differential that the functionT(t,x) =e 4tsin(2x) satisfies theone-space dimensional heat equationTt= :We first computeTt,Tt= 4e tsin(2x).
10 Now computeTxx,Tx= 2e tcos(2x) Txx= 4e tsin(2x)ThereforeTt= primer on differential that the functionf(t,x) = (vt x)3, withv R, satisfiesthe one-space dimensional wave equationftt= :We first computeftt,ft= 3v(vt x)2 ftt= 6v2(vt x).Now computefxx,fx= 3(vt x)2 fxx= 6(vt x).Thereforeftt= primer on differential that every functionf(t,x) =u(vt x), withv Randu:R Rtwice continuously differentiable, satisfies the one-spacedimensional wave equationftt= :We first computeftt,ft=v u (vt x) ftt=v2u (vt x).Now computefxx,fx= u (vt x)2 fxx=u (vt x).