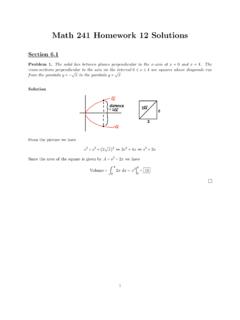
SOLUTIONS OF SOME HOMEWORK PROBLEMS Problem set 1

1 SOLUTIONS OF SOME HOMEWORK PROBLEMSMATH 114 Problem set 14. LetD4denote the group of symmetries of a square. Find the order ofD4andlist all normal subgroups 8 elements:1, r, r2, r3,d1, d2, b1, b2,whereris the rotation on 90 ,d1, d2are flips about diagonals,b1, b2are flips aboutthe lines joining the centers of opposite sides of a square. LetNbe a normal subgroupofD4. Note thatd1=rd2r 1,b1=rb2r 1,d1d2=b1b2= ifd1 N, thend2 N, similarly forb1, b2. Note thatd1b1=r. Thus, ifNcontains a flip andN6=G, thenNeither containsd1, d2or containsb1, b2. LetNcontaind1andd2, thenN={1, d1, d2, r2}. In the same way ifNcontainsb1andb2,thenN={1, b1, b2, r2}. Finally, ifNdoes not contain flips, thenN={1, r, r2, r3}orN={1, r2}.

2 Thus,D4have one 2-element normal subgroup and three 4-elementsubgroups. Then, as always, there are normal subgroups {1} Show that then-cycle (1.. n) and the transposition (12) generate the per-mutation groupSn, every element ofSncan be written as a product of (12),u= (1.. n). It is easy to check thatusu 1= (23) ,u2su 2= (34), .. , un 2su2 n= (n 1, n).Thus, any subgroup ofSnwhich containsuandsmust contain all adjacent trans-positions. But the adjacent transpositions generateSn. Find a cyclic subgroup of maximal order order ofs Snequals the least common multiple of the lengthsof the cycles ofs. Forn= 8, the possible cycle lengths are less than 9. By simplecheck we see that a product of disjoint 3-cycle and 5-cycle has the maximal order a maximal cyclic group set 21.

3 Anautomorphismof a groupGis an isomorphism fromGto itself. Denote byAutGthe set of all automorphisms ofG.(a) Prove that AutGis a group with respect to the operation of : February 22, OF SOME HOMEWORK PROBLEMS math 114(b) LetGbe a finite cyclic group. Describe AutG.(c) Give an example of an abelianGsuch that AutGis not part (a) is a straightforward check. For (b) letG=Zn. If AutG, then is determined by (1), as (k) = (1 + + 1) = (1) + + (1) =k (1).It is easy to check now that is injective if and only if (1) andnare relativelyprime. LetZ n={s|0< s < n,(s, n) = 1},with operation of multiplication modn. The map AutZn Z ngiven by 7 (1)is an do (c) letG=Z2 Z2. Then AutGis isomorphic toS3because any permutationof (1,0), (0,1) and (1,1) defines an automorphism Use the same notations as in Problem 1.

4 Let gbe the map ofGto itself definedby g(x) =gxg 1, hereg G.(a) Show that g AutG.(b) Let InnG={ g|g G}. Show that InnGis a normal subgroup in (a) g(xy) =gxyg 1=gxg 1gyg 1= g(x) g(y).(b) First check that InnGis a subgroup g h(x) =g(hxh 1)g 1= (gh)x(gh) 1= gh(x).To check that InnGis normal let AutG, then g 1(x) = (g 1(x)g 1)= (g) ( 1(x)) (g 1)= (g)x (g 1).Thus, g 1= (g).4. One makes necklaces from black and white beads. Letpbe a prime necklaces are the same if one can be obtained from anotherby a rotation or aflip over. How many different necklaces ofpbeads one can make? group acting on the necklaces isDp. We have to find the numberof orbits. Possible subgroups ofDpare groups generated by one flip or the cyclicsubgroup of rotations, as follows from the fact that|Dp|= 2pand the all rotations preserve a necklace, then its beads are all of the same color.

5 In thiscase the stabilizer is the wholeDp, and we have exactly two such a stabilizer of a necklace is a flip. Then the necklace is symmetric. We canchoose color ofp+12beads, the other can be obtained by the symmetry. Thus, wehave exactly 2p+12 2 orbits. (We subtract 2 because necklaces with all beads of thesame color are already counted). Each orbit haspnecklaces in it. All other necklacesSOLUTIONS OF SOME HOMEWORK PROBLEMSMATH 1143do not have any symmetry. To count their number we must subtract the number ofnecklaces which we already counted from 2p. That gives2p p(2p+12 2) orbit with a trivial stabilizer has 2pelements. The number of such orbits is2p p(2p+12 2) 22p=2p 1 1p 2p 12+ total number of orbits is2p 1 1p 2p 12+ 1 + 2p+12=2p 1 1p+ 2p 12+ Assume thatNis a normal subgroup of a groupG.

6 Prove that ifNandG/Naresolvable, thenGis Consider the seriesN N1 {1},K K1 {1},such thatKi/Ki+1andNj/Nj+1are abelian. Letp:G Kdenote the naturalprojection. Then for the seriesG p 1(K1) N N1 {1}p 1(Ki)/p 1(Ki+1) =Ki/Ki+1by the second isomorphism theorem. Thus,Gis For any permutationsdenote byF(s) the number of fixed points ofs(kis afixed point ifs(k) =k). LetNbe a normal subgroup ofAn. Choose a non-identicalpermutations Nwith maximal possibleF(s).(a) Prove that any disjoint cycle ofshas length not greater than 3. (Hint: ifs N, thengsg 1 Nfor any even permutationg).(b) Prove that the number of disjoint cycles insis not greater than 2.(c) Assume thatn 5. Prove thatsis a 3-cycle.

7 (d) Use (c) to show thatAnissimpleforn 5, not have propernon-trivial normal subgroups . ( Hint:Anis generated by 3-cycles, as it was provenin class).. ckandc1be one of the longest cycles. Assume that thelength ofc1is greater than 3. Letc1= (x1, x2, .. , xl) ,u= (x1, x2, x3).Thensus 1u 1= (x1, x4, x2) (sus 1u 1) = 3< F(s). Contradiction. That proves (a).4 SOLUTIONS OF SOME HOMEWORK PROBLEMS math 114 Assume now thatk 3. Since all cycles ofshave the length 2 or 3. One can findtwo cycles of the same length. Sayc1= (a, b, c),c2= (d, e, f).Letu= (b, c) (e, f). Thens 1usu 1=c1c2 (s 1usu 1)< F(s). Contradiction. If we assume thatc1= (a, b),c2= (c, d),putu= (a, b, c). Thens 1usu 1= (a, c) (b, d) obtainF(s 1usu 1)< F(s).

8 Contradiction. Hence (b) is letn 5. Assume thatsis not a 3-cycle. Thens=c1c2,wherec1andc2are either both transpositions or both 3-cycles. First, assume thatc1andc2are both transpositions. In this casec1= (a, b),c2= (c, d).Sincen 5, there ise6=a, b, c, d. Letu= (a, b, e). Thens 1usu 1= (b, e, a),againF(s 1usu 1) = 3<4 =F(s). Finally, letc1= (a, b, c)(d, e, f).Play the same game withu= (b, c, e). Getsus 1u 1= (a, f, c, b, e).Obtain contradiction one 3-cycle, thenNmust contain all 3-cycles, because all 3-cyclesare conjugate inAnforn 5. ThereforeN=An. set 32. Ifpis prime andpdivides|G|, thenGhas an element of Sylow theoremGhas a subgroupPof orderpn. Letg P. Thenthe order ofgispk, and the order ofgpk Letpandqbe prime andq6 1 modp.

9 If|G|=pnq, thenGis the second Sylow theorem there is only one it byP. ThenPis normal sincegP g 1=Pfor anyg G. As we proved inclassPis solvable, the quotientG/Pis solvable. HenceGis solvable by Problem 5homework Suppose that|G|<60 and|G|= 2m3n. Check thatGis solvable. Hint: proveby induction on|G|. First, show that the number of Sylow 2- subgroups is 3 or theSOLUTIONS OF SOME HOMEWORK PROBLEMSMATH 1145number of Sylow 3- subgroups is 4. Then construct a homomorphismf:G S3orS4. By induction the kernel and the image offare solvable. HenceGis , assume thatm 3. The number of Sylow 3- subgroups is 1 or4 by the second Sylow theorem. If it is 1, proceed as in Problem3. If it is 4, thenGacts on the 4-element set of Sylow 3- subgroups by conjugation.

10 Thus, we have anon-trivial homomorphismf:G S4. Imfis solvable as a subgroup of a solvablegroup, Kerfis solvable by induction assumption. HenceGis letm 4. Recall that|G|<60. The casen= 0 is known. Thereforem= 4, n= 1,|G|= 48. The number of Sylow 2- subgroups is 1 or 3. If it is 1 wecan proceed as in Problem 3. If it is 3, thenGacts on the 3-element set of Sylow2- subgroups , there is a non-trivial homomorphismf:G S3and we can finish theargument as in the previous Show that any group of order less than 60 is solvable. Hint: use the previousproblems to eliminate most of numbers below the maximal prime factor of|G|. First, assume thatp > |G|=pk, withk < p. Then the number of Sylowp- subgroups ofGis 1, andwe can go to the quotient and proceed by induction on|G|.