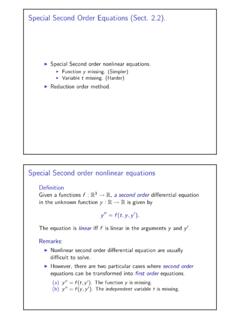
Theorem 1. The Fundamental Theorem of Calculus Part 1

1 THE Fundamental Theorem OF CALCULUSJOHN D. this note, we give a different proof of the Fundamental Theoremof Calculus part 2 than that given in Thomas Calculus , 11th Edition, Thomas,Weir, Hass, Giordano, ISBN-10: 0321185587, Addison-Wesley,c 2005. Wealso discuss the extent to which the Fundamental Theorem of Calculus part 2implies the Fundamental Theorem of Calculus part Fundamental Theorem of Calculus part 1We recall the Fundamental Theorem of Calculus part 1, hereafter referred toas part 1, with a slight revision from the formulation in Thomas Calculus , 11thEdition, Thomas, Weir, Hass, Giordano, ISBN-10.

2 0321185587, Addison-Wesley,c 2005, hereafter referred to as Thomas Fundamental Theorem of Calculus part 1 Iffis contin-uous on[a,b]andFis the function on[a,b]defined by the rule:(1)F(x) = xaf(t)dt,thenFis continuous on[a,b]and an antiderivative forfon(a,b); that is to say:(2)F (x) =ddx( xaf(t)dt)=f(x).for allx (a,b). part 1 has the following important corollary concerning the existence of of AntiderivativesIffis continuous on[a,b], thenthere exists a continuous functionFon[a,b]such thatFis an antiderivative forfon(a,b).

3 Is an immediate consequence of part 1 which gives one such functionF; namely the functionFon [a,b] defined by the ruleF(x) = xaf(t)dt. Date: December 1, Subject :12J. Fundamental Theorem of Calculus part 2We recall the Fundamental Theorem of Calculus part 2, hereafter referred to asPart 2, with a slight revision from the formulation in Thomas Fundamental Theorem of Calculus part 2 Iffis continu-ous on[a,b]andFis a continuous function on[a,b]such thatFis an antiderivativeforfon(a,b), then:(3) baf(x)dx=F(x) (b) F(a).

4 In Thomas Calculus , part 2 is proved by first proving part 1 without using Part2 and then deducing part 2 from part 1. In this section, we shall prove part 2without using part Alternative Proof of part 2 without using part 1 Sincefis continuous on [a,b], it follows from Theorem 1 of Section of Thomas Calculus thatfis integrable on [a,b]; that is to say, baf(x) be a positive real number. By the definition of the definite integral as alimit of Riemann sums, it follows that there exists a positive real number suchthat ifP:a=x0<.

5 < xn=bis any partition of [a,b] with||P||< andci [xi 1,xi],1 i n, and:(4) i=1f(ci)( (x))i,then:(5) R baf(x)dx < .Now choose a positive integernsuch that .= (b a)/n < . Letxi=a+i ,1 i n. ThenP:a=x0< .. < xn=bis a partition of [a,b], ( (x)) xi 1= ,1 i nand||P||= max{( (x))i|1 i n}= < .Letibe an integer such that 1 i n. SinceFis continuous on [a,b] and[xi 1,xi] is contained in [a,b],Fis continuous on [xi 1,xi]. Likewise, sinceFisdifferentiable on (a,b) and (xi 1,xi) is contained in (a,b),Fis differentiable on(xi 1,xi).

6 It follows from the Mean Value Theorem for Derivatives that there existsci (xi 1,xi) such that:(6)F(xi) F(xi 1)xi xi 1=F (ci).SinceFis an antiderivative forfon (a,b) andci (xi 1,xi) (a,b), it followsthatF (ci) =f(ci). Hence, by equation (6) and the fact thatxi xi 1= ( (x))i,it follows that:THE Fundamental Theorem OF CALCULUS3(7)F(xi) F(xi 1)( (x))i=f(ci)which implies that:(8)F(xi) F(xi 1) =f(ci)( (x)) equation (8) holds for each integeriwith 1 i n, it follows that:(9) i=1f(ci)( (x))i=n i=1(F(xi) F(xi 1))= (F(x1) F(x0)) + (F(x2) F(x1)) +.

7 + (F(xn 1) F(xn 2)) + (F(xn) F(xn 1))= F(x0) +F(xn) =F(xn) F(x0) =F(b) F(a).Since||P||< , It follows from equations (5) and (9) that:(10) (F(b) F(a)) baf(x)dx < .Since equation (10) holds for every positive real number , it follows that(11) baf(x)dx=F(b) F(a). Relationship of Parts 1 and 2As mentioned earlier, Thomas Calculus proves part 2 by first proving part 1and then deducing part 2 from part in Section 2 we proved part 2 without using part 1, this raises the question,can we deduce part 1 from part 2?

8 The following Theorem shows that the answer to this question is affirmative,provided that we have Corollary 2 on the existence of antiderivatives. Since theproof of Corollary 2 depends upon part 1, this Theorem falls short of demonstratingthat part 2 implies part Fundamental Theorem of Calculus part 2 ( Theorem 3) andCorollary 2 on the existence of antiderivatives imply the Fundamental Theorem ofCalculus part 1 ( Theorem 1). part 2 and Corollary 2 and suppose thatfis continuous on [a,b].

9 By Corollary 2, there exists a continuous functionGon [a,b] such thatGis differ-entiable on (a,b) andGis an antiderivative forfon (a,b).Suppose thatx [a,b]; that is to say,a x , on the one hand, thatx=a. ThenF(x).= xaf(t)dt= aaf(t)dt= 0andG(x) G(a) =G(a) G(a) = 0. Hence:4J. MCCARTHY(12)F(x) = xaf(t)dt=G(x) G(a).Suppose, on the other hand, thatx6=a. Thena < x b. It follows that[a,x] [a,b] and (a,x) (a,b). Hence, sincefis continuous on [a,b],fis contin-uous on [a,x]; sinceGis continuous on [a,b],Gis continuous on [a,x]; sinceGisdifferentiable on (a,b),Gis differentiable on (a,x); and sinceGis an antiderivativeforfon (a,b),Gis an antiderivative forfon (a,x).

10 It follows from part 2 that:(13)F(x) = xaf(t)dt=G(x) G(a).Hence, by equations (12) and (13), for allx [a,b]:(14)F(x) =G(x) G(a).SinceGis continuous on [a,b] and constant functions are continuous and adifference of continuous functions is continuous, it follows from equation (14) thatFis continuous on [a,b].SinceGis an antiderivative forfon (a,b), it follows from equation (14), thedifference rule for derivatives, and the constant rule for derivatives that:(15)F (x) = (G(x) G(a)) =G (x) 0 =f(x) 0 =f(x)for allx (a,b).