A pendulum with a moving support point

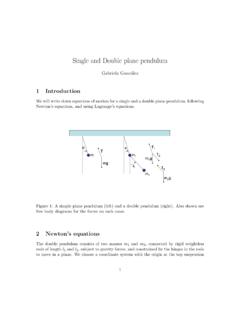
1 A pendulum with a moving support pointGabriela Gonz alezSeptember 12, 2006 Consider a pendulum with massmhanging from a rod of lengthl. The support pointmoves horizontally with a known functionR(t) =X(t) i+Y(t) j. We can use the angle between the vertical and the pendulum rod as a generalized coordinate, the only oneneeded to describe the position vector of the massmisr=R+l(sin i+ cos j)The velocity isv= R+l (cos i sin j)The kinetic energy isT=12mv2=12m(| R|2+l2 2+ 2l ( Xcos Ysin )The potential energy isV= mg r= mgcos mgYand although time dependent (throughY(t), it is not dissipative, since it doesn t contain .The Lagrangian isL=T VL( , ,t) =12ml2 2+ml ( X(t) cos Y(t) sin ) +12m( X(t)2+ Y(t)2) +mglcos +mgY(t)Lagrange s equation is0 =ddt L L =ddt(ml2 +ml( Xcos Ysin )) (ml ( Xsin Ycos ) mglsin )=ml2 +ml( Xcos Ysin ) +mglsin =ml2 +ml(g Y) sin +ml Xcos 1We see that the equation of motion is unchanged if R= 0: this is because the support -would be moving with constant velocity, and thus it is just like setting up the system inanother inertial frame.))

2 We also notice that a vertical acceleration of the support point islike increasing or decreasing the local gravitational accelerationg: this can be recognizedas the relativity principle (imagine Einstein in an elevator).The energy function ish= L L= (ml2 +ml( Xcos Ysin )) (12ml2 2+ml ( Xcos Ysin ) +12mV2+mglcos +mgY)=12ml2 2 mglcos 12mV2 mgYThe first two terms are the usual expression for the energy of a pendulum hanging froma fixed support (or the energy with respect to a frame moving with the support ), but thereare extra the Lagrangian is not homogeneous in the second order with respect to (throughthe terms X, Y), the energy function is not equal to the mechanical energy, which wecan calculate:E=T+V=12ml2 2+12mV2+ml ( Xcos Ysin ) mglcos mgY=h+ml ( Xcos Ysin ) +mV2We can calculate the time derivative of the energy from this expression.

3 E=12ml2 2+12mV2+ml ( Xcos Ysin ) mglcos mgYdEdt=ml2 +m R R+ml ( Xcos Ysin ) +ml ( Xcos Ysin ) ml 2( Xsin + Ycos ) +mgl sin mg Y= ml ( Xcos Ysin +gsin ) +m R R+ml ( Xcos Ysin ) +ml ( Xcos Ysin ) ml 2( Xsin + Ycos ) +mgl sin mg Y=m R R+ml ( Xcos Ysin ) ml 2( Xsin + Ycos ) mg Ywhere we have used Lagrange s equation l = Xcos Ysin +gsin to obtain the the Lagrangian depends explicitly on time, we know that the energy function isnot conserved:dhdt= L t= ml ( Xcos + Ysin ) m R R mg YWe can also use this result to calculate the rate of change in the mechanical energy:E=h+ml ( Xcos Ysin ) +mV2dEdt=dhdt+ddt(ml ( Xcos Ysin ) +mV2)= mg Y+ml ( Xcos Ysin ) ml 2( Xsin + Ycos ) +m R Rwhich is of course the same expression we had obtained us look at some particular cases: Small angle approximation.

4 1 The Lagrangian isL=12ml2 2+ml ( Xcos Ysin ) +12m( X2+ Y2) +mglcos +mgY 12ml2 2 12mgl 2+ml ( X Y ) +12mV2+mglThe equation of motion isddt L L =ml2 +ml( X Y ) +mgl 0 =l + X+ (g Y) The equation of motion does not necessarily with periodic solutions, unless X= 0and Yis constant, in which case the oscillations have a frequency 2= (g Y) frequency increases if the motion is accelerated down (in the same direction thangravity), or lower if the support is accelerated energy isE= =12ml2 2+12mV2+ml ( Xcos Ysin ) mglcos mgY 12ml2 2+12mgl 2+ml ( X Y ) +12mV2 mgY mglThe rate of change in energy isdEdt= mg Y+ml ( Xcos Ysin ) ml 2( Xsin + Ycos ) +m R R l X mg Y+m R R3 Uniform horizontal motion : X=V, Y= 0 This is like a pendulum inside a car moving with uniform velocity on a horizontalroad.

5 The equation of motion is not changed from that of a simple pendulum , but theenergy is not constant. This is because there is a force of the vehicle on the pendulum ,reacting to the motion of the pendulum itself. Small oscillations of the pendulumhave the same frequency 2=g/l, and the energy changedE/dt lV = mgV is also an oscillating function with the same frequency. Uniform vertical motion : X= 0, Y=VThis is like a pendulum moving in an elevator moving with uniform velocity. Theequations of motion are unchanged, but the energy is not constant. Small oscillationshave the same frequency 2=g/l, but nowdE/dt mgV: there is a constantincrease or decrease of energy, depending on whether the support is going up (andenergy is increasing), or down (and energy is decreasing).

6 This can also be interpretedas the change in gravitational potential energy due to the vertical motion . Horizontal periodic motion :X=X0cos( t),Y= 0 The equation of motion for small oscillations isl +g = 2X0cos( t). This is adriven oscillation, and after initial transients, the pendulum will oscillate with thesame frequency as the support point : = 0cos( t), with amplitude 0given bythe equation of motion :l +g = 2X0cos( t)( l 2+g) 0= 2X0 0=X0 2g l 2=X0l 2 20 2with 20=g/l. The horizontal displacement of the pendulum with respect to theinertial frame isx=X+l . The ratio of amplitude of the horizontal motion of thependulum mass,x, to the amplitude of the horizontal motion of the top,X0, is calledthe transfer function (a function of driving frequency) of the pendulum to supportmotion:F( ) =xX0=X0+l 0=X0(1 + 2 20 2)=X0 20 20 2If driven at low frequencies ( 2 g/l), the pendulum will follow the support , witha small angle , ansx X: the rod is almost vertical (although the maximumpendulum displacement is larger than the top s maximum displacement).

7 At drivingfrequencies near the natural pendulum frequency, 0, the motion will grow verylarge: the system is near resonance . At driving frequencies higher than 0, thependulum motion is out of phase with respect to the motion of the support , since4the transfer function is negative, and the maximum pendulum displacement is nowsmaller than the top s maximum displacement. At very high driving frequencies( 0), the limit of the transfer function is zero, and the angular amplitude ofthe pendulum motion isX0/l: the pendulum mass stays near the equilibrium point ,while the top moves back and energy (for small oscillations) isE=12ml2 2+12mgl 2+ml X+12mV2=12ml(l 2+g) 20cos2( t) +ml 2 0X0sin2( t) +12m 2X20cos2( t)=12mX20(cos2( t)(( 2+ 20) 2 20 2+ 2)+ sin2( 2t) 2 2 20 2)and the power absorbed by the system isdEdt=mlV +m R R=mlX0 0 2sin tcos t+mX20 2sin tcos t=12mX20 2( 2 20 2+ 1)sin 2 t=12mX20 2 20 20 2sin 2 tSincedE/dtis periodic with frequency 2 , the system absorbs energy during half ofthe cycle of the top motion , and returns the energy in the other half.

8 Which half iswhich depends on the driving frequency being larger or smaller than the pendulumenergy, , the pendulum moving in phase or out of phase with the support . The peakpower is proportional to a factorX20 20 2/( 20 2): this is very large near resonance;small and proportional toX0 2at low driving frequencies; and independent of drivingfrequency at large driving frequencies, X20 20. Vertical periodic motion :Y=Y0cos( t),X= equation of motion is l =l(g Y) sin =l(g+ 2Y0cos t) sin . The motionof the support adds an oscillating component to the gravitational acceleration. Thisis not a driven oscillator like the previous case, because the oscillatory driving forceis vertical but the natural oscillatory pendulum motion is horizontal. IfY0 2 g,we can use a perturbative approach to find a solution starting with the pendulumsolution.

9 IfY0 2 g, we can also use a perturbative approach to find a solutionstarting with the driven pendulum energy isE=12ml2 2+12mV2+ml ( Xcos Ysin ) mglcos mgY=12ml2 2 mglcos +12mY20 2cos2 t+mlY0 sin cos t mgY0cos tand the power absorbed isdEdt= mg Y+ml ( Xcos Ysin ) ml 2( Xsin + Ycos ) +m R R=mgY0 sin t+mlY0 sin tsin +mlY0 2sin tcos +mY20 2sin tcos t=mgY0 sin t+12mY20 2sin 2 t+mlY0 sin t( sin + 2cos ) support point in uniform circular motionThe support will move withX(t) =Rsin t,Y(t) =Rcos 2+ml ( X(t) cos Y(t) sin ) +12m( X(t)2+ Y(t)2) +mglcos +mgY(t)=12ml2 2+mglcos +mlR cos( t ) +12mR2 2+mgRcos tLagrange s equation is0 =ddt L L =ml2 +mglsin mlR 2sin( t )=ml2 +ml(g+R 2cos t) sin mlR 2cos sin tFor small oscillations, we see that there is an oscillating contribution to the restoringgravitational force, and an oscillatory driving energy isE=12ml2 2 mglcos +mlR sin( t ) +12mR2 2 mgRcos tand the power absorbed by the system isdEdt= mg Y+ml ( Xcos Ysin ) ml 2( Xsin + Ycos ) +m R R=mgR sin t+mlR cos( t ) +mlR 2 sin( t )6