Chapter 2 Kinematics

1 Chapter 2 KinematicsQuestion 2 1A bugBcrawls radially outward at constant speedv0from the center of a ro-tating disk as shown in Fig. P2-1. Knowing that the disk rotates about its cen-terOwith constant absolute angular velocity relative to the ground (wherek k = ), determine the velocity and acceleration of the bug as viewed by anobserver fixed to the Figure P2-1 Solution to Question 2 1 For this problem it is convenient to choose a fixed reference frameFand a non-inertial reference frameAthat is fixed in the disk. Corresponding to referenceframeFwe choose the following coordinate system:Origin at PointOEx=AlongOBat Timet=0Ez=Out of PageEy=Ez Ex2 Chapter 2. KinematicsCorresponding to the reference frameAthat is fixed in the disk, we choose thefollowing coordinate systemOrigin at PointOex=AlongOBez=Out of Page(=Ez)ey=ez exThe position of the bug is then resolved in the basis{ex,ey,ez}asr=rex( )Now, since the platform rotates about theez-direction relative to the ground,the angular velocity of reference frameAin reference frameFis given asF A= ez( )The velocity is found by applying the basic kinematic givesFv=Fdrdt=Adrdt+F A r( )Now we haveAdrdt= rex=v0ex( )F A r= ez rex= rey( )Adding Eqs.

2 ( ) and ( ), we obtain the velocity of the bug in reference frameFasFv=v0ex+ rey( )The acceleration is found by applying the basic kinematic equation toFv. ThisgivesFa=Fddt Fv =Addt Fv +F A Fv( )UsingFvfrom Eq. ( ) and noting thatv0and are constant, we have thatAddt Fv = rey= v0eyF A Fv= ez [v0ex+r ey]= 2rex+ v0ey( )Therefore, the acceleration in reference frameFis given asFa= 2rex+2 v0ey( )3 Question 2 2A particle, denoted byP, slides on a circular table as shown in Fig. P2-2. Theposition of the particle is known in terms of the radiusrmeasured from thecenter of the table at pointOand the angle where is measured relative to thedirection ofOQwhereQis a point on the circumference of the table. Knowingthat the table rotates with constant angular rate , determine the velocity andacceleration of the particle as viewed by an observer in a fixed reference Figure P2-2 Solution to Question 2 2 For this problem it is convenient to define a fixed inertial reference frameFand two non-inertial reference framesAandB.

3 The first non-inertial referenceframeAis fixed to the disk while the second non-inertial reference frameBisfixed to the direction ofOP. Corresponding to the fixed inertial reference frameF, we choose the following coordinate system:Origin at pointOEx=AlongOxatt=0Ez=Out of PageEy=Ez ExCorresponding to non-inertial reference frameA, we choose the following co-ordinate system:Origin at pointOex=AlongOQez=Out of Page(=Ez)ey=ez ex4 Chapter 2. KinematicsFinally, corresponding to reference frameB, we choose the following coordinatesystem:Origin at pointOer=AlongOPez=Out of Pagee =ez erThen, the position of the particle can be desribed in terms of the basis{er,e ,ez}asr=rer.( )Now, in order to compute the velocity of the particle, it is necessary to applythe basic kinematic equation. In this case since we are interested in motionas viewed by an observer in the fixed inertial reference frameF, we need todetermine the angular velocity ofBinF. First, sinceArotates relative toFwith angular velocity , we have thatF A= = ez( )Next, sinceBrotates relative toAwith angular rate about theez-direction,we have thatA B= ez( )Then, applying the theorem of addition of angular velocities,we have thatF B=F A+A B= ez+ ez=( + )ez( )The velocity in reference frame is then found by applying the rate of changetransport theorem asFv=Fdrdt=Bdrdt+F B r( )Now we haveBdrdt= rer( )F B r=( + )ez rer=r ( + )e ( )Adding Eqs.

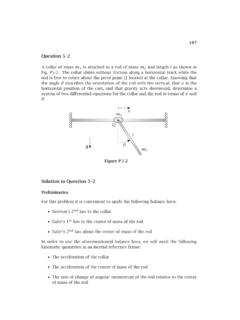
4 ( ) and ( ), we obtain the velocity of the particlein referenceframeFasFv= rer+r ( + )e ( )The acceleration is found by applying the rate of change transport theorem toFv. This givesFa=Fddt Fv =Bddt Fv +F B Fv( )5 UsingFvfrom Eq. ( ) and noting again that is constant, we haveBddt Fv = rer+h r ( + )+r ie ( )F B Fv=( + )ez h rer+r ( + )e i= r ( + )2er+ r ( + )e ( )Adding Eqs. ( ) and ( ), we obtain the acceleration of the particle in refer-ence frameFasFa=h r r ( + )2ier+hr +2 r ( + )ie ( )6 Chapter 2. KinematicsQuestion 2 3A collar slides along a rod as shown in Fig. P2-3. The rod is freeto rotate abouta hinge at the fixed pointO. Simultaneously, the rod rotates about the verticaldirection with constant angular velocity relative to the ground. Knowing thatrdescribes the location of the collar along the rod, that is the angle measuredfrom the vertical, and that = k k, determine the velocity and acceleration ofthe collar as viewed by an observer fixed to the Figure P2-3 Solution to Question 2 3 First, letFbe a fixed reference frame.

5 Then, choose the following coordinatesystem fixed in reference frameF:Origin at pointOEx=Along Ez=Orthogonal to Plane ofShaft and Arm att=0Ey=Ez ExNext, letAbe a reference frame fixed to the vertical shaft. Then, choose thefollowing coordinate system fixed in reference frameA:Origin at pointOex=Along ez=Orthogonal to Plane ofShaft and Armey=ez ex7 Finally, letBbe a reference frame fixed to the rod. Then, choose the followingcoordinate system fixed in reference frameB:Origin at pointOer=AlongOPez=uze =ez erThe geometry of the bases{ex,ey,ez}and{er,e ,ez}is shown in Fig. 2-1. UsingFig. 2-1, the relationship between the basis{ex,ey,ez}and{er,e ,ez}is givenasex=cos er sin e ey=sin er+cos e ( ) ere exeyez Figure 2-1 Geometry of Bases{ex,ey,ez}and{er,e ,ez}for Question 2 position of the particle can then be expressed in the basis{er,e ,ez}asr=rer( )Now, since{er,e ,ez}is fixed in reference frameB, and we are interested inobtaining the velocity and acceleration as viewed by an observer fixed in theground ( , reference frameF), we need to obtain an expression for the angularvelocity of reference frameBin reference frameF.

6 First, since reference frameArotates relative to reference frameFwith angular velocity and lies alongtheex-direction, we have thatF A= = ex( )Next, since reference frameBrotates relative to reference frameAwith angularrate about theez-direction. Therefore,A B= ez( )8 Chapter 2. KinematicsThen, using the angular velocity addition theorem, we have the angular velocityof reference frameBin reference frameFasF B=F A+A B= ex+ ez( )Now, since we have determined that the position of the collar is expressed mostconveniently in terms of the basis{er,e ,ez}, it is also most convenient to ex-pressF Bin terms of the basis{er,e ,ez}. In particular, substituting the ex-pression forexfrom Eq. ( ) into Eq. ( ), we obtainF BasF B= (cos er sin e )+ ez= cos er sin e + ez( )The velocity in reference frameFis then found by applying the rate of changetransport theorem between reference framesBandFasFv=Fdrdt=Bdrdt+F B r( )Now we have thatBdrdt= rer( )F B r=( cos er sin e + ez) rer= rsin Ez+r e ( )Adding Eq.

7 ( ) and Eq. ( ), we obtain the velocity of the collar in referenceframeFasFv= rer+r e +r sin ez( )The acceleration of the collar is then obtained by applying the rate of changetransport theorem toFvbetween reference framesBandFasFa=Fddt Fv =Bddt Fv +F B Fv( )Now we haveBddt Fv = rer+( r +r )e +h ( rsin +r cos )iez( )F B Fv=( cos er sin e + ez) ( rer+r e +r sin ez)=r cos ez r 2cos sin e + r sin ez r 2sin2 er+ r e r 2er= (r 2+r 2sin2 )er+( r r 2cos sin )e +(r cos + r sin )ez( )Adding Eqs. ( ) and ( ), we obtain the acceleration of the collar in refer-ence frameFasFa=( r r 2 r 2sin2 )er+(2 r +r r 2cos sin )e +2 ( rsin +r cos )ez( )9 Question 2 4A particle slides along a track in the form of a parabolay=x2/aas shownin Fig. P2-4. The parabola rotates about the vertical with a constant angularvelocity relative to a fixed reference frame (where = k k). Determine thevelocity and acceleration of the particle as viewed by an observer ina fixedreference Figure P2-4 Solution to Question 2 4 For this problem it is convenient to define a fixed inertial reference frameFand a non-inertial reference frameA.

8 Corresponding to reference frameF, wechoose the following coordinate system:Origin at PointOEx=AlongOQWhent=0Ey=AlongOyWhent=0 Ez=Ex EyFurthermore, corresponding to reference frameA, we choose the following co-ordinate system:Origin at PointOex=AlongOQey=AlongOyez=ex eyThe position of the particle is then given in terms of the basis{ex,ey,ez}asr=xex+yey=xex+(x2/a)ey( )Furthermore, since the parabola spins about theey-direction, the angular ve-locity of reference frameAin reference frameFis given asF A= = ey( )10 Chapter 2. KinematicsThe velocity in reference frameFis then found using the rate of change trans-port theorem asFv=Fdrdt=Adrdt+F A r( )Usingrfrom Eq. ( ) andF Afrom Eq. ( ), we haveAdrdt= xex+(2x x/a)ey( )F A r= ey (xex+(x2/a)ey)= xez( )Adding Eqs. ( ) and ( ), we obtainFvasFv= xex+(2x x/a)ey xez( )The acceleration in reference frameFis found by applying the rate of changetransport theorem toFvasFa=Fddt Fv =Addt Fv +F A Fv( )UsingFvfrom Eq.

9 ( ) andF Afrom Eq. ( ), we haveAddt Fv = xex+h2( x2+x x)/aiey xez( )F A Fv= ey ( xex+(2x x/a)ey xez)= xez 2xex( )Adding Eq. ( ) and ( ), we obtainFaasFa=( x 2x)ex+h2( x2+x x)/aiey 2 xez( )11 Question 2 5A satellite is in motion over the Earth as shown in Fig. P2-5. The Earth is mod-eled as a sphere of radiusRthat rotates with constant angular velocity ina directionezwhereezlies along a radial line that lies in the direction fromthe center of the Earth at pointOto the North Pole of the Earth at , the center of the Earth is assumed to be an absolutelyfixed position of the satellite is known in terms of anEarth-centered Earth-fixedCartesian coordinate system whose right-handed basis{ex,ey,ez}is defined asfollows: The directionexlies orthogonal toezin the equatorial plane of the Earthalong the line fromOtoPwherePlies at the intersection of the equatorwith the great circle called thePrime Meridian The directioneylies orthogonal to bothexandezin the equatorial planeof the Earth such thatey=ez exUsing the basis{ex,ey,ez}to express all quantities, determine the velocity andacceleration of the spacecraft (a) as viewed by an observer fixed to the Earth and(b) as viewed by an observer in a fixed inertial reference frame.

10 Prime MeridianSpacecraftEquatorexeyezrNOPQ Figure P2-5 Solution to Question 2 5 First, letFbe a fixed inertial reference frame. Next, letAbe a reference framethat is fixed in the planet. Corresponding to reference frameA, we choose the12 Chapter 2. Kinematicsfollowing coordinate system:Origin at pointOex=AlongOPez=AlongONey=ez ex(=AlongOQ)The position of the spacecraft is then given in terms of the basis{ex,ey,ez}asr=xex+yey+zez( )Now, since the planet rotates with constant angular velocity about theON-direction relative to reference frameF, we have thatF A= ez( )The velocity of the spacecraft is then found by applying the rate of changetransport theorem asFv=Fdrdt=Adrdt+F A r( )Now we haveAdrdt= xex+ yey+ zez( )F A r= ez (xex+yey+zez)= xey yex( )Adding Eqs. ( ) and ( ), we obtainFvasFv=( x y)ex+( y+ x)ey+ zez( )Next, the acceleration of the spacecraft in reference frameFis found by apply-ing the rate of change transport theorem toFvasFa=Fddt Fv =Addt Fv +F A Fv( )Now we haveAddt Fv =( x y)ex+( y+ x)ey+ zez( )F A Fv= ez [( x y)ex+( y+ x)ey+ zez]= ( x y)ey ( y+ x)ex( )Adding Eqs.