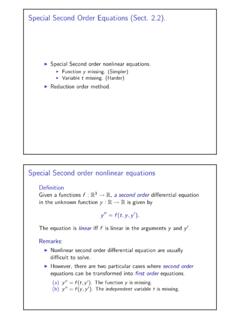
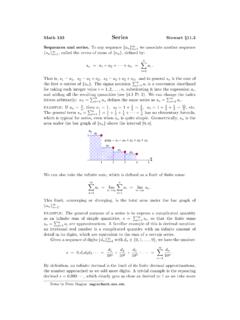
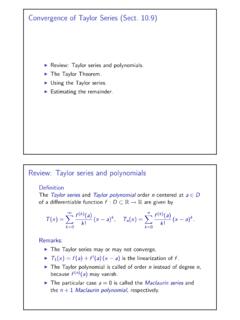
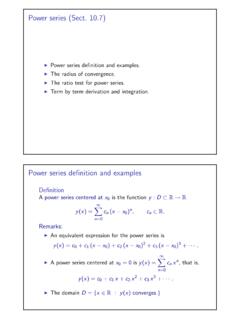
Transcription of Convolution solutions (Sect. 6.6). - users.math.msu.edu
1 Convolution solutions (Sect. ).IConvolution of two of Transform of a response decomposition solutions (Sect. ).IConvolution of two of Transform of a response decomposition of two piecewise continuous functionsf,g:R Risthe functionf g:R Rgiven by(f g)(t) = t0f( )g(t )d .Remarks:If gis also called the generalized product definition of Convolution of two functions also holds inthe case that one of the functions is a generalized function,like Dirac s of two piecewise continuous functionsf,g:R Risthe functionf g:R Rgiven by(f g)(t) = t0f( )g(t )d .Remarks:If gis also called the generalized product definition of Convolution of two functions also holds inthe case that one of the functions is a generalized function,like Dirac s of two piecewise continuous functionsf,g:R Risthe functionf g:R Rgiven by(f g)(t) = t0f( )g(t )d .Remarks:If gis also called the generalized product definition of Convolution of two functions also holds inthe case that one of the functions is a generalized function,like Dirac s of two the Convolution off(t) =e tandg(t) = sin(t).
2 Solution:By definition: (f g)(t) = t0e sin(t )d .Integrate by parts twice: t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0 t0e sin(t )d ,2 t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0,2(f g)(t) =e t cos(t) 0 + sin(t).We conclude:(f g)(t) =12[e t+ sin(t) cos(t)].CConvolution of two the Convolution off(t) =e tandg(t) = sin(t).Solution:By definition: (f g)(t) = t0e sin(t )d .Integrate by parts twice: t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0 t0e sin(t )d ,2 t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0,2(f g)(t) =e t cos(t) 0 + sin(t).We conclude:(f g)(t) =12[e t+ sin(t) cos(t)].CConvolution of two the Convolution off(t) =e tandg(t) = sin(t).Solution:By definition: (f g)(t) = t0e sin(t )d .Integrate by parts twice: t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0 t0e sin(t )d ,2 t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0,2(f g)(t) =e t cos(t) 0 + sin(t).We conclude:(f g)(t) =12[e t+ sin(t) cos(t)].
3 CConvolution of two the Convolution off(t) =e tandg(t) = sin(t).Solution:By definition: (f g)(t) = t0e sin(t )d .Integrate by parts twice: t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0 t0e sin(t )d ,2 t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0,2(f g)(t) =e t cos(t) 0 + sin(t).We conclude:(f g)(t) =12[e t+ sin(t) cos(t)].CConvolution of two the Convolution off(t) =e tandg(t) = sin(t).Solution:By definition: (f g)(t) = t0e sin(t )d .Integrate by parts twice: t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0 t0e sin(t )d ,2 t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0,2(f g)(t) =e t cos(t) 0 + sin(t).We conclude:(f g)(t) =12[e t+ sin(t) cos(t)].CConvolution of two the Convolution off(t) =e tandg(t) = sin(t).Solution:By definition: (f g)(t) = t0e sin(t )d .Integrate by parts twice: t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0 t0e sin(t )d ,2 t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0,2(f g)(t) =e t cos(t) 0 + sin(t).
4 We conclude:(f g)(t) =12[e t+ sin(t) cos(t)].CConvolution of two the Convolution off(t) =e tandg(t) = sin(t).Solution:By definition: (f g)(t) = t0e sin(t )d .Integrate by parts twice: t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0 t0e sin(t )d ,2 t0e sin(t )d =[e cos(t )] t0 [e sin(t )] t0,2(f g)(t) =e t cos(t) 0 + sin(t).We conclude:(f g)(t) =12[e t+ sin(t) cos(t)].CConvolution solutions (Sect. ).IConvolution of two of Transform of a response decomposition of (Properties)For every piecewise continuous functions f , g, and h, hold:(i)Commutativity:f g=g f;(ii)Associativity:f (g h) = (f g) h;(iii)Distributivity:f (g+h) =f g+f h;(iv)Neutral element:f 0 = 0;(v)Identity element:f = :(v): (f )(t) = t0f( ) (t )d =f(t).Properties of (Properties)For every piecewise continuous functions f , g, and h, hold:(i)Commutativity:f g=g f;(ii)Associativity:f (g h) = (f g) h;(iii)Distributivity:f (g+h) =f g+f h;(iv)Neutral element:f 0 = 0;(v)Identity element:f = :(v): (f )(t) = t0f( ) (t )d =f(t).
5 Properties of (Properties)For every piecewise continuous functions f , g, and h, hold:(i)Commutativity:f g=g f;(ii)Associativity:f (g h) = (f g) h;(iii)Distributivity:f (g+h) =f g+f h;(iv)Neutral element:f 0 = 0;(v)Identity element:f = :(v): (f )(t) = t0f( ) (t )d =f(t).Properties of :(1): Commutativity:f g=g definition of Convolution is,(f g)(t) = t0f( )g(t )d .Change the integration variable: =t ,henced = d ,(f g)(t) = 0tf(t )g( )( 1)d (f g)(t) = t0g( )f(t )d We conclude:(f g)(t) = (g f)(t).Properties of :(1): Commutativity:f g=g definition of Convolution is,(f g)(t) = t0f( )g(t )d .Change the integration variable: =t ,henced = d ,(f g)(t) = 0tf(t )g( )( 1)d (f g)(t) = t0g( )f(t )d We conclude:(f g)(t) = (g f)(t).Properties of :(1): Commutativity:f g=g definition of Convolution is,(f g)(t) = t0f( )g(t )d .Change the integration variable: =t ,henced = d ,(f g)(t) = 0tf(t )g( )( 1)d (f g)(t) = t0g( )f(t )d We conclude:(f g)(t) = (g f)(t).
6 Properties of :(1): Commutativity:f g=g definition of Convolution is,(f g)(t) = t0f( )g(t )d .Change the integration variable: =t ,henced = d ,(f g)(t) = 0tf(t )g( )( 1)d (f g)(t) = t0g( )f(t )d We conclude:(f g)(t) = (g f)(t).Properties of :(1): Commutativity:f g=g definition of Convolution is,(f g)(t) = t0f( )g(t )d .Change the integration variable: =t ,henced = d ,(f g)(t) = 0tf(t )g( )( 1)d (f g)(t) = t0g( )f(t )d We conclude:(f g)(t) = (g f)(t).Properties of :(1): Commutativity:f g=g definition of Convolution is,(f g)(t) = t0f( )g(t )d .Change the integration variable: =t ,henced = d ,(f g)(t) = 0tf(t )g( )( 1)d (f g)(t) = t0g( )f(t )d We conclude:(f g)(t) = (g f)(t).Properties of :(1): Commutativity:f g=g definition of Convolution is,(f g)(t) = t0f( )g(t )d .Change the integration variable: =t ,henced = d ,(f g)(t) = 0tf(t )g( )( 1)d (f g)(t) = t0g( )f(t )d We conclude:(f g)(t) = (g f)(t).
7 Convolution solutions (Sect. ).IConvolution of two of Transform of a response decomposition Transform of a (Laplace Transform)Iff,ghave well-defined Laplace TransformsL[f],L[g], thenL[f g] =L[f]L[g].Proof:The key step is to interchange two start wethe product of the Laplace transforms,L[f]L[g] =[ 0e stf(t)dt] [ 0e s tg( t)d t],L[f]L[g] = 0e s tg( t)( 0e stf(t)dt)d t,L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d Transform of a (Laplace Transform)Iff,ghave well-defined Laplace TransformsL[f],L[g], thenL[f g] =L[f]L[g].Proof:The key step is to interchange two start wethe product of the Laplace transforms,L[f]L[g] =[ 0e stf(t)dt] [ 0e s tg( t)d t],L[f]L[g] = 0e s tg( t)( 0e stf(t)dt)d t,L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d Transform of a (Laplace Transform)Iff,ghave well-defined Laplace TransformsL[f],L[g], thenL[f g] =L[f]L[g].Proof:The key step is to interchange two start wethe product of the Laplace transforms,L[f]L[g] =[ 0e stf(t)dt] [ 0e s tg( t)d t],L[f]L[g] = 0e s tg( t)( 0e stf(t)dt)d t,L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d Transform of a (Laplace Transform)Iff,ghave well-defined Laplace TransformsL[f],L[g], thenL[f g] =L[f]L[g].
8 Proof:The key step is to interchange two start wethe product of the Laplace transforms,L[f]L[g] =[ 0e stf(t)dt] [ 0e s tg( t)d t],L[f]L[g] = 0e s tg( t)( 0e stf(t)dt)d t,L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d Transform of a (Laplace Transform)Iff,ghave well-defined Laplace TransformsL[f],L[g], thenL[f g] =L[f]L[g].Proof:The key step is to interchange two start wethe product of the Laplace transforms,L[f]L[g] =[ 0e stf(t)dt] [ 0e s tg( t)d t],L[f]L[g] = 0e s tg( t)( 0e stf(t)dt)d t,L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d Transform of a :Recall:L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d variables: =t+ t,henced =dt;L[f]L[g] = 0g( t)( te s f( t)d )d [f]L[g] = 0 te s g( t)f( t)d d key step: Switch the order of = tautaut0L[f]L[g] = 0 0e s g( t)f( t)d t d .Laplace Transform of a :Recall:L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d variables: =t+ t,henced =dt;L[f]L[g] = 0g( t)( te s f( t)d )d [f]L[g] = 0 te s g( t)f( t)d d key step: Switch the order of = tautaut0L[f]L[g] = 0 0e s g( t)f( t)d t d.
9 Laplace Transform of a :Recall:L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d variables: =t+ t,henced =dt;L[f]L[g] = 0g( t)( te s f( t)d )d [f]L[g] = 0 te s g( t)f( t)d d key step: Switch the order of = tautaut0L[f]L[g] = 0 0e s g( t)f( t)d t d .Laplace Transform of a :Recall:L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d variables: =t+ t,henced =dt;L[f]L[g] = 0g( t)( te s f( t)d )d [f]L[g] = 0 te s g( t)f( t)d d key step: Switch the order of = tautaut0L[f]L[g] = 0 0e s g( t)f( t)d t d .Laplace Transform of a :Recall:L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d variables: =t+ t,henced =dt;L[f]L[g] = 0g( t)( te s f( t)d )d [f]L[g] = 0 te s g( t)f( t)d d key step: Switch the order of = tautaut0L[f]L[g] = 0 0e s g( t)f( t)d t d .Laplace Transform of a :Recall:L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d variables: =t+ t,henced =dt;L[f]L[g] = 0g( t)( te s f( t)d )d [f]L[g] = 0 te s g( t)f( t)d d key step: Switch the order of = tautaut0L[f]L[g] = 0 0e s g( t)f( t)d t d.
10 Laplace Transform of a :Recall:L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d variables: =t+ t,henced =dt;L[f]L[g] = 0g( t)( te s f( t)d )d [f]L[g] = 0 te s g( t)f( t)d d key step: Switch the order of = tautaut0L[f]L[g] = 0 0e s g( t)f( t)d t d .Laplace Transform of a :Recall:L[f]L[g] = 0g( t)( 0e s(t+ t)f(t)dt)d variables: =t+ t,henced =dt;L[f]L[g] = 0g( t)( te s f( t)d )d [f]L[g] = 0 te s g( t)f( t)d d key step: Switch the order of = tautaut0L[f]L[g] = 0 0e s g( t)f( t)d t d .Laplace Transform of a :Recall:L[f]L[g] = 0 0e s g( t)f( t)d t d .Then, is straightforward to check thatL[f]L[g] = 0e s ( 0g( t)f( t)d t)d ,L[f]L[g] = 0e s (g f)( )d L[f]L[g] =L[g f]We conclude:L[f g] =L[f]L[g].Laplace Transform of a :Recall:L[f]L[g] = 0 0e s g( t)f( t)d t d .Then, is straightforward to check thatL[f]L[g] = 0e s ( 0g( t)f( t)d t)d ,L[f]L[g] = 0e s (g f)( )d L[f]L[g] =L[g f]We conclude:L[f g] =L[f]L[g].