Fluids – Lecture 3 Notes - MIT

1 Fluids Lecture 3 Notes1. 2-D Aerodynamic Forces and Moments2. Center of Pressure3. Nondimensional CoefficientsReading: Anderson Forces and MomentsSurface force distributionThe fluid flowing about a body exerts a local force/area (or stress)~fon each point of thebody. Its normal and tangential components are the pressurepand the shear stress .( magnitude greatly exaggerated)Vff RMRLDNA resultant force, and moment about ref. pointalternative components of resultant forceplocal pressure and shear stress componentsforce/area distribution on airfoil rdsrThe figure above greatly exaggerates the magnitude of the stress component just to makeit visible. In typical aerodynamic situations, the pressurep(or even the relative pressurep p ) is typically greater than by at least two orders of magnitude, and so~fis verynearly perpendicular to the surface.

2 But the small often significantly contributes to drag,so it cannot be neglected stress distribution~fintegrated over the surface produces a resultant force~R, and alsoa momentMabout some chosen moment-reference point. In 2-D cases, thesign conventionforMis positive nose up, as shown in the componentsThe resultant force~Rhas perpendicular components along any chosen axes. These axes arearbitrary, but two particular choices are most useful in Axes: The~Rcomponents are the dragDand the liftL, parallel and perpendic-ular to~V .Body Axes: The~Rcomponents are the axial forceAand normal forceN, parallel andperpendicular to the airfoil chord one set of components is computed, the other set can then beobtained by a simple axistransformation using the angle of attack . Specifically,LandDare obtained fromNandAas Asin D=Nsin +Acos Force and moment calculationA cylindrical wing section of chordcand spanbhas force componentsAandN, and mo-mentM.

3 In 2-D it s more convenient to work with the unit-span quantities, with the spandimension divided A/bN N/bM M/bV u up (s )p (s )ll u u (s )x l(s )ly usudsslldsu upudsbcOn the upper surface, the unit-span force components actingon an elemental area of widthdsuaredN u= ( pucos usin )dsudA u= ( pusin + ucos )dsuAnd on the lower surface they aredN = (p cos sin )ds dA = (p sin + cos )ds Integration from the leading edge to the trailing edge points produces the total = TELEdN u+ TELEdN A = TELEdA u+ TELEdA 2 The moment about the origin (leading edge in this case) is theintegral of these forces,weighted by their moment armsxandy, with appropriate LE= TELE x dN u+ TELE x dN + TELEy dA u+ TELEy dA From the geometry, we havedscos =dxdssin = dy= dydxdxwhich allows all the above integrals to be performed inx, using the upper and lower shapesof the airfoilyu(x) andy (x).

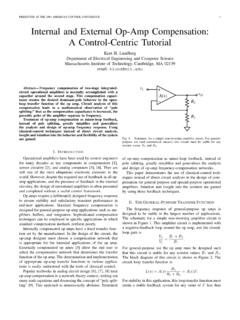
4 Anderson has the complete practice, the shear stress has negligible contributions to the lift and moment, givingthefollowing simplified = cos c0(p pu)dx+ sin c0(p dy dx pudyudx)dxM LE= c0[pu(x+dyudxyu) p (x+dy dxy )]dxA somewhat less accurate but still common simplification is to neglect the sin term inL ,and thedy/dxterms inM .L c0(p pu)dxM LE c0 (p pu)x dxThe shear stress cannotbe neglected when computing the dragD on streamline bodiessuch as airfoils. This is because for such bodies the integrated contributions ofptowardD tend to mostly cancel, leaving the small contribution of quite of PressureDefinitionThe value of the momentM depends on the choice of reference point. Using the simplifiedform of theMLEintegral, the momentMreffor an arbitrary reference pointxrefisM ref= c0 (p pu) (x xref)dx=M LE+L xrefThis can be positive, zero, or negative, depending on wherexrefis chosen, as illustrated inthe one particular reference locationxcp, called thecenter of pressure , the moment is definedto be cp=M LE+L xcp 0xcp= M LE/L 3M < 0M = 0ororxcplp puLLLM > 0 The center of pressure asymptotes to + or as the lift tends to zero.

5 This awkwardsituation can easily occur in practice, so the center of pressure is rarely used in reasons which will become apparent when airfoil theory is studied, it is advantageousto define the standard location for the moment reference point of an airfoil to be at itsquarter-chord location, orxref=c/4. The corresponding standard moment is usually writtenwithout any c/4 M = c0 (p pu) (x c/4)dxAerodynamic ConventionsAs implied above, the aerodynamicist has the option of picking any reference point for themoment. The lift and the moment then represent the integratedpl pudistribution. Considertwo possible representations:1. A resultant liftL acts at the center of pressurex=xcp. The moment about thispoint is zero by definition:M cp= 0. Thexcplocation moves with angle of attack in acomplicated A resultant liftL acts at the fixed quarter-chord pointx=c/4.

6 The moment aboutthis point is in general nonzero:M c/46= figure shows how theL ,M , andxcpchange with angle of attack for a typical camberedairfoil. Note that with representation 1, thexcplocation moves off the airfoil and tends to+ asL approaches zero. Fixing the moment reference point, as in representation 2, is asimpler and preferable approach. Choosing the quarter-chord location for this is especiallyattractive, sinceM then shows little or no dependence on the angle of attack. This surprisingfact will come from a more detailed airfoil analysis later inthe puxcpxcpxcpc/4c/4c/4 = 5 = 0 = 5L (M =0)L M CoefficientsThe forces and moment depend on a large number of geometric and flow parameters. Itis often advantageous to work with nondimensionalized forces and moment, for which mostof these parameter dependencies are scaled out.

7 For this purpose we define the followingreference parameters:Reference area:SReference length: Dynamic pressure :q =12 V2 The choices forSand are arbitrary, and depend on the type of body involved. For aircraft,traditional choices are the wing area forS, and the wing chord or wing span for . Thenondimensional force and moment coefficients are then definedas follows:Lift coefficient:CL Lq SDrag coefficient:CD Dq SMoment coefficient:CM Mq S For 2-D bodies such as airfoils, the appropriate reference area/span is simply the chordc, andthe reference length is the chord as well. Thelocalcoefficients are then defined as Lift coefficient:c L q cLocal Drag coefficient:cd D q cLocal Moment coefficient:cm M q c2 These local coefficients are defined for each spanwise location on a wing, and may vary acrossthe span. In contrast, theCL,CD,CMare single numbers which apply to the whole