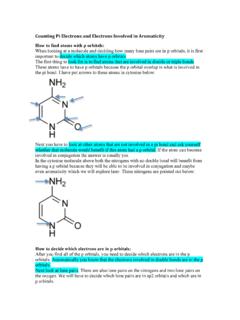
Transcription of The Early History of Pi
1 The Early History of PiEgyptIn 1856 a Scotsman by the name of Henry Rhind purchased apapyrus in Egypt. A copy dating to 1,650 , it consisted of a collectionof mathematics problems prepared for a typical mathematics student of of algebra, linear systems, mensuration, and mathematical amuse-ments were assembled. There was no formal theory, just the one problem wefind the statement:A square of side 8 has the area of a circle of diameter this statement, the student was expected to compute the area of anycircle. Fortunately, the concept of proportion was well understood and thusby scaling any circular area was easily , using the fact that the true area of a circle of diameter 9 r2= ( )2 the area of a square of side 8 is 64, we easily see that theeffectivevaluefor is or 31381 Most modern histories give this value to the Bible: Also he made a molten sea of ten cubits from brim to brim, round incompass, andfive cubits the height thereof; and a line of thirty cubits didcompass it round about.
2 Old Testament, 1 Kings 7:23 BabylonThe Baylonians enjoyed a sophisticated society. Located in theMesopotamian River Valley, they created large monuments to their gods andkings while engaging in commerce throughout the do this they required some considerable mathematical skills. Excellentrecords of their whole society, including their mathematics has been preservedin the tens of thousands of clay ..For example, they seemed to be well aware of the Pythagorean theorema thousand years before Pythagoras. They developed a method of squareroot extraction equivalent to Newton s method. Generally, they used theapproximation = , at Susa (about 200-300 miles from Babylon) a new set of tabletswere unearthed in 1936. These tablet were on whole concerned with theratios of areas and perimeters of regular polygons to their respective sidelengths. For example, wefindThe ratio of the perimeter of a regular inscribedhexagon to the circumference of the circle in sex-agesimal numbers is0; 57,36 = 0 +5760+35602.
3 9600 Thecorrectvalueofthisratiois62 and this gives aneffectivevalue of to Greeks, living in an open society free of political and othersocial restraints, were thefirst to develop the axiomatic method andrigorin their problems vexed (and blessed) their mathematical development: How to square the circle. How to double the cube. How to trisect an they didn t know is that thefirst problem depends on thevery nature of .What they didn t know was that these problems would require more thantwo milleniato Euclid sElements,BookXII,Proposition2,wefind the following result.(It s proof is based on the Eudoxus Method of Exhaustion, the Greek versionof taking limits, without formally defining them.)The ratio of the areas of two circles is as the ratio of thesquares upon their this we know that the Greek mathematicians were well aware thatthere must be a mathematical model equivalent to the one we know asA= r2 ArchimedesThe greatest mathematician of antiquity, Archimedes(287-212 ), proved two fundamental relations about the perimeters andareas of inscribed and circumscribed regular polygons.
4 With respect to acircle of radiusr,letb1= an inscribed hexagon with perimeterp1and areaa1B1= an circumscribed hexagon with perimeterP1and areaA1 Further, the regular inscribed 6 2npolygons,similarly, the circumscribed following formulae give the relations between the perimeters and areasof these 6 +1=2pnPnpn+Pnpn+1=ppnPn+1an+1=panAnAn+1= 2an+1 Anan+1+AnUsingn-gonsupto96sideshederives 31071< <317 Following Archemedes,Apollonius(250-175 ), in his book, now lost,on rapid calculation techniques,Quick Delivery,approximates as the last great mathematicians of antiquity. His book,On Conics,didforconics what Euclid did for plane great astronomer and the father of trigonometryClaudius Ptolemy(100-178 ) gives 377120= his method of derivation is unknown. His book, commonly knownasAlmagest, was studied and considered the source of trigonometry up tothe time of Copernicus (fl. 1500)RomeThe Romans were warriors, engineers, authors, and rulers.
5 Theywere not mathematicians. Even with all the Greek mathematics available,they computed circular areas using proportion and the statementA wheel of diameter 4 feet has a perimeter gives the approximation 318 ChinaGenerally, the Chinese, even before 100 , used 3. Theyalso used the alternative approximation, 10 By the 5thcenturymore accurate approximations were known. Tsu Ch ung-Chih gives two setsof approximationsinexact 227accurate 355113= also < < that to eleven decimal places = of this accu-racy were not achieved in the West until the best approximation to was given in about 500 by Aryabhata using the following descriptionAdd 4 to 100, multiply by 8, and add 62, result is approximately the circumference ofa circle of which the diameter is 20, course this gives(100+4)8+62,00020,000=31771250= , the Hindu approximation to was usually 833 , the great arab mathematicianAl-Khw arizm i,thefather of algebra, uses two approximations for :317and =62,83220,000= about 1436Al-Kashigives2 ,sixteen decimal places, which is prodigiously accurate for the Modern History of PiFranceThe French mathematicianFrancois Vieta(1540-1603), thefirstmathematician to us symbolic notation for knowns (consonants) and un-knowns (vowels) discovered thefirstdirectformulafor the calculation of.
6 2=1q12r12+12q12s12+12r12+12q12 This is easily derived beginning with an inscribed square, and successivelydoubling the number of sides, getting next the octagon, the 16-gon, .. Usingthe notationanfor the area of the inscribedn-gon, apply the formulaa2n=ansec nrecursively, beginning withn= 4 (the square). Calculating thusly, Vietaobtained ten places Ludolph van Ceulen(1540-1610) computed accurate to20 places. Later, he computed to 31 places. So proud was he of thisaccomplishment that he had the digits engraved on his tombstone. At thistime is sometimes calledLudolph sconstant. (It is not yet called .)The known accuracy of now exceeds anycomputational number of mathematical developments come from Eng-land. Thefirst approximation to came from the clergyman, cryptogra-pher and mathematicianJohn Wallis(1616-1703). Using a complicatedinterpolation-like argument based on the quadratures of the functions (1 +x)n,n=0,.., he produced2 =1 3 3 5 5 7 2 2 4 4 6 6 From thisLord William Brouncker(1620?)
7 -1684), thefirst president ofthe Royal Society, discovered the formula4 =1+12+92+252+492+ James Gregory(1637-1675) derived the well known (to us) formulaZx0dt1+t2=arctanx=x x33+x55 x77+ ,now called Gregory s formula. Evaluating atx=1,weget 4=1+13 15+17 This series was used byAbraham Sharp(1651-1742) under instructionsfrom Edmund Halley (1656-1743) (of comet fame) to calculate to 72 places.(Note the convergence is very slow. How many terms are required for 10 72accuracy?)John Machin(1680-1751), a professor of astronomy at Gresham Collegein London obtained 100 places of accuracy for the computation of usingGregory s formula 4= 4 arctan15 arctan1239 EvenIssac Newtonin 1665-1666 calculated from the formula =3 34+24(112 15 25 128 27+ )=3 34+24Z140px x2dxSaid Newton: I am ashamed to tell you to how manyfigures I carried these computations,having not other business at the time. In 1874, Machin s formula was used byWilliam Shanks(1812-1882) toachieve 707 places of accuracy for.
8 Because the series is alternating andalmost geometric, the convergence is very, very fast. Only about 510 termsare required to achieve this the greatest mathematician that ever lived,Leon-hard Euler(1707-1783), was born in Basel and studied under James eventually found a position at St. Petersburg, originally to serve on thefaculty of medicine and 1730, due in part to the death of his colleague and friend, Nicolas Bernoulli,he found himself in the chair of natural philosophy not medicine. His con-tributions to mathematics have been well documented and are also contributed to the derivation of formulas with .In 1779 he determined the formula =20arctan17 8arctan379from which the computation of can be made using Gregory s , Euler derived a different formula for the arc tangent as well. Healso derived the formulas 26= Xn=11n2 490= Xn=11n4 6945= Xn=11n6 89,450= Xn=11n8 1093,955= Xn= well as formulas for the sum of the remaining reciprocal (even) integersup to to order coined the modern symbol for pi, , after John Wallis whofirst used for pi.
9 In 1801 he also used the modern symbols foreandi= proved the most important relation in all of mathematics:ei +1=0 TheNatureofPiJohann Lambert(1728-1777) was born in the Alsace region, the son of apoor tailor. Managing to teach himself elementary mathematics and pursuehis mathematical studies while a tutor for a Swiss family, he eventually wasable to enjoy the company of the day s greatest mathematicians includingLagrange and Euler. In 1761 he proved thatifxis rational (and non-zero), then neitherexnor tanxcan be proves this using a continued fraction expansion ofegiven by Euler this time there were so manycircle squarersthat in 1775 theParis Academy passed a resolution thatno moresolutions on the quadratureofthecircleshouldbeexaminedbyi tsofficials. This resolution applied aswell to angle trisections and the doubling of the cube. The conviction wasgrowing that the solution of squaring the circle was can be shown that by using a straight edge and compass, the only magni-tudes that can be constructed from whole magnitudes are numbers involvingsquare roots, square roots of square roots, etc.
10 For example, 2,q3+ 4,r5+q6+ 7, numbers are also among the solutions of all polynomial equations withrational coefficients. The set of all solutions, real and complex, of polynomialequations with rational coefficients are called thealgebraic numbers are were discovered byJoseph Liouville(1809-1882)in 1844 with the number110+1102!+1103!+ In 1873,Charles Hermite(1822-1901) proved thatewas transcendentalby showing that it cannot be the solution of a polynomial equation withrational , in 1882, Lindemann(1852-1939) proved that is transcen-dental by showing that ifa, b, c,..andm, n, r,..are algebraic numbersthe the equationaem+ben+cer+ =0has no rational solutions. Thus the equationeix+1=0cannot subsist ifxis algebraic. Using Euler s celebrated formulaei +1=0it follows that is not algebraic. The circle cannot be squared!Bill No. 246, 1897. State of Indiana Be it enacted by the General Assembly of the State of Indiana: It has beenfound that the circular area is to the quadrant of the circumference, as thearea of an equilateral rectangle is to the square on one side.